Kepler at first tried to fit Mars orbit into the scheme that Copernicus had cooked up and found large errors (over a degree) between Copernicus systems predictions for Mars, and the actual position in the sky as measured by Brahe. He tried reintroducing the equant of Ptolemy for both the deferent and the epicycle of Mars, since Copernicus had taught that the planetary epicycle was just the same as the orbit of the sun. But no matter what he did, he kept getting disagreement with Brahe's observations. At one point he had reduced the difference to about 8 arc minutes, an almost impossible small angle to see, but he felt that Brahe had been such a good observer that this could not be an observational error.
The structure of Ptolemy's equant was that it had three special points, the location of the earth (in Ptolemy) or of the sun (in Copernicus's system), the centre of the circle which defined the deferent and the location of the equant about this the uniform motion occurred. But from early Greek work, predating Hipparchus, and extensively studied by Aristarchus and by Archimedes, there was another figure closely related to the circle, the ellipse. Both were "conic sections" obtained by cutting a circular cone. The circle is obtained by cutting perpendicular to the axis of the cone, the ellipse by cutting it at a slant.
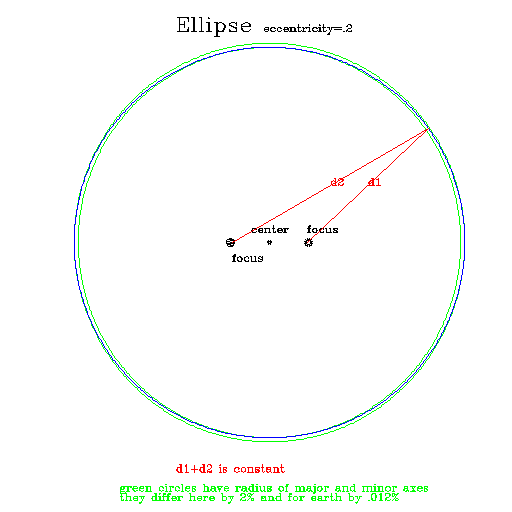
The ellipse is an ovoid figure, with three special points arrayed along the
long axis of the ellipse. There is the centre of the ellipse, the intersection of
the two axis of symmetry (if you fold the ellipse along those lines, the two
halves lie directly on top of each other). Along the longer axis of the
ellipse are two special points-- the foci of the ellipse. These have the
property that if you were to coat the ellipse so as to make it a mirror for
light rays travelling in the plane of the ellipse, all of the light rays
leaving one of the foci would be focused on the other one. It is more or less
defined by the property that the distance from one of the foci to any point
on the ellipse, plus the distance from the other focus to that same point is a
constant. Ie, you draw an ellipse by lying a loop of string so it loops around
two small pillars at the foci, placing a pencil into the loop and moving the
pencil so that the string is tight.

This ellipse is drawn for an eccentricity (distance between the two foci divided by the distance from one side of the figure to the other along the line through the two foci) of .2 This is larger than the eccentricity of any of the planets except Mercury, and is done so you can see the effect that the eccentricity has. The green circles are drawn with the same centre and with radii equal to the maximum distance of the ellipse from the centre, and the minimum. Note the similarity to the Ptolemy's orbit, which also has two points (the earth and the equant) but has the orbit being a circle instead of an ovoid around the centre. For small eccentricity (less than .05) the two figures are essentially indistinguishable.
So, Ptolemy had a figure which had a centre of symmetry for the figure, and had two special points, the earth and the equant, equidistant from the centre. The difference was that the ellipse was not a circle (but became more and more like a circle as the two foci came closer and closer together). Perhaps an ellipse would work better than Ptolemy's circle. The one focus could be the location of the earth or sun, and the other focus would play a role something like an equant. How would the planet move around this figure? One could demand, a la Ptolemy, that the planet would move uniformly (equal angles in equal times) as seen from the other focus, as Ptolemy had it. But this did not work either. But for small separation of the foci (small eccentricity) he found that Ptolemy's rule had another feature, namely that the area of the triangle swept out by the line from the focus where the earth or sun resided was the same for equal times. (for small times, the line from the earth/sun focus to the ellipse sweeps out a good approximation to a triangle. The area of that triangle is half the base times the perpendicular height from that base line to the focus. The "equal areas" motion, and the "uniform rotation about the equant" produced the same motion along the ellipse if the the eccentricity of the ellipse (the ratio between the distance between the foci to the largest diameter of the ellipse) was very small. Perhaps this was the law of motion! It had the huge advantage that it was a law expressed entirely with respect to the occupied focus of the ellipse, instead of being expressed in term of the unoccupied focus (the equant). Surely it makes more sense for God to have created the laws of the universe referring to actual things rather than to imaginary points. When he tried this for Mars, the difference between the observations of Brahe and the motion predicted by the theory disappeared. This led him to formulate two new laws based on this
Applying this to the other planets and Brahe's observations of them, the law fit (except for the moon for which the behaviour, as had already been recognized by Hipparchus, was so messy, it was hard to figure out any simple law for its motion.)
In addition, since Copernicus had shown that his identification of the epicycle with the sun's orbit (Ptolemy)/earth's orbit( new Heliocentric model), this also told us what the relative size of the orbits of the various planets were, and since Brahe had carefully measured the period of the planets with respect to the fixed stars, Kepler found another relation
These three laws have come to be called Kepler's laws. They were the culmination of the efforts to describe the heavens that had begun with the early Geeks. Note that the goal of these efforts had changed. For the Babylonians, the importance of the motions was astrological-- how did the Heavens affect human activities? Furthermore, the most important heavenly activities, the eclipses, has been downplayed by Kepler. The moon was too complicated in its motion (which of course plays crucial role in Astrology). It is however interesting that Kepler himself made money by casting horoscopes, and his mother had almost been executed as a witch. Ie, astrology was far from dead.
But the heavens were ruled by law. Why these laws and not others, noone understood. Furthermore the old laws of motion of the heavens, uniform motion on circles, which had been strained by Ptolemy, lay completely broken but had been replaced by other, more complicated laws. Uniform motion on circles is easy to understant. Equal areas motion on an ellipse (of some arbitrary eccentricity) is harder to understand.
The story from the early Greeks to Kepler is an astonishing story of accomplishment, and set the tone for how Science in general should be done. The tradition married strong theoretical traditions and speculation with superbly careful observation. It was not sufficient that one simply theorize about the world, as the early Greek philosophers tended to do. (As my son has said, the problem with tracing things back to the Greeks is that one can find almost any position on the nature of the world one desires in some philosopher or another). It was not sufficient to make careful observations of the world and to look for patterns in those observations, as the Babylonians did in the heavens. It took the union of the two, the application of theory to the observations, and the willingness to alter the theory if the observations demanded it. It of course helped that the theory, circles with uniform motion, was a pretty good approximation to the facts. Had the solar system been like most solar systems around other stars, with eccentricies more like .2 to .5 or even higher, the job would have been far more difficult.
The initial theory did not fit perfectly. Hipparchus was willing to insert off-centre locations for the earth, and to discuss the possibility of circular orbits about empty points. Ptolemy went further and allowed uniform motion not about the empty centre of circles but about other empty points (the equant and the point on the deferent).
Kepler was willing to abandon 2000 years of circular dogma, to introduce ellipses and "equal area" instead of "equal angle" motion as the nature of Uniform motion. This interplay of careful observation with opportunistic alteration of the theory in the face of the "facts" even if those facts were at the borders of the accuracy with which they could be obtained is what has driven all areas of science, and it got its start in this strand of physics which was the study of the heavens. It was its huge success which sparked the possibility of huge successes in all areas of the study of the world.
While Galileo has largely been given the credit for the "scientific method" it was much older than him and certainly goes back to Hipparchus and Ptolemy. Even if on looks at "doing experiments" rather than "doing observations" it may be that his father beat him out, but that is another story which we will get to in the "harmony" thread of this course. .
copyright W Unruh (2018)